Back to . . . 

Curve Bank Home
Please be patient. These animations require dowloading two QuickTime movies. The speed may depend on your internet connection.

NCB  Deposit #5 by Aarnout Brombacher of Cape Town, South Africa.
 

NCB  Deposit #6 also the contribution of Aarnout Brombacher.

The National Curve Bank gratefully thanks Aarnout Brombacher for permission to use his wonderful animations. 
This work was completed while he was a graduate student working with Dr. James Wilson at the University of Georgia, Athens.
Today Brombacher teaches in Cape Town and has headed the Mathematics Teachers of South Africa professional organization.

The essential cycloid equations:

Many famous mathematicians
have investigated the cycloid.

Def:  The cycloid is the locus of a point on the circumference of a circle
where a circle of radius a rolls along a fixed straight line.

With national rivalries and individual competition among the most distinguished of mathematicians, the cycloid has been called the

"Helen of Geometers" - the most beautiful curve in the world.
No topic in mathematics has a more outstanding list of investigators.  Indeed, those who have published on the cycloid and its related curves constitute a virtual "Who's Who" of mathematics.

Galileo (1599) apparently named the curve and attempted to find its area by weighing various pieces of metal slices representing the rolling disc.  His student Torricelli, as well as Fermat, Roberval, and Descartes all published articles on finding its exact area.  Roberval and Sir Christopher Wren, the great British architect, succeeded in calculating the length of the arc.  In 1658 Pascal offered a prize for the solution to a number of problems of "la Roulette."  Wallis entered the competition, but apparently Pascal never awarded the prize.

The mechanically minded were also fascinated.  Gear teeth were proposed by Desargues (1630s) for a cycloid as it rolled along its fixed straight line.  The first pendulum clock, invented by Huygens, contained a device for making the pendulum "isochronous" - equal in length of time - by using the cycloidal arc and the evolute of the cycloid as a guide.

Knowing the brachistochrone was related to the cycloid, James (Johann) Bernoulli (1698) challenged others to investigate its properties.  Leibniz, Newton, Jakob Bernoulli and L'Hospital all accepted this challenge by publishing solutions.

It is fun to guess how Galileo, Torricelli, Fermat, Roberval, Descartes, Wren, Pascal, Wallis, Desargues, Huygens, two Bernoullis, Leibniz, Newton and L'Hospital would have reacted if they had been able to see how easily contemporary students can display and animate "their" cycloid.

 
Great Cycloid Links
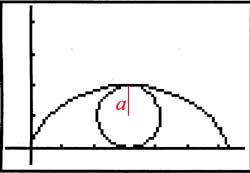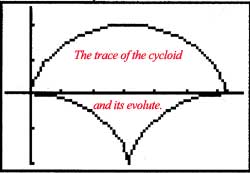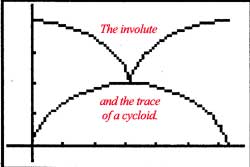From Brombacher's student project:
http://jwilson.coe.uga.edu/EMT668/EMT668.Student.Folders/BrombacherAarnout/EMT669/cycloids/cycloids.html

Aarnout Brombacher was a graduate student from South Africa working with Dr. James Wilson at the University of Georgia, Athens.  He is now a teacher in Cape Town and President of the Mathematics Teachers of South Africa.
 
The NCB thanks the Huntington Library, San Marino, California, for permission to reproduce this text as it appears in the first edition of Herman Melville's American classic, Moby Dick.

Melville wrote that his time at sea had served as his "Harvard" and his "Yale."  He seemed anxious to prove that he had learned mathematics to the extent that he was familiar with the properties of the cycloid.

Click on Melville's picture to view a larger image.