| Back to . .
. .
 Curve Bank Home Curve Bank Home
Dr. Paul Chabot
Department of Mathematics
California State University
Los Angeles
|

The Cycloid Family of Curves
Cycloid, Trochoid, Epicycloid,
Hypocycloid, Epitrochoid and Hypotrochoid
Create Your Own Animations Using Maple!
|
| The graphics in this deposit were created using Maple
software.

|
NCB Deposit # 30
|
A
Sampler for the Student . . . .
Each animation in the left
column will repeat twice.
|
These are
very large files.
Be patient! |
Cycloid
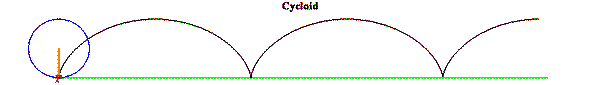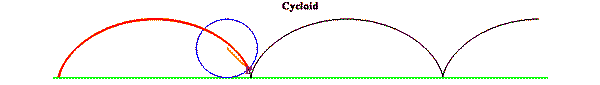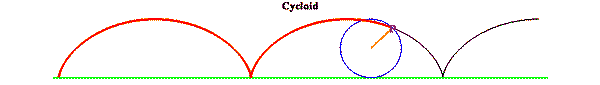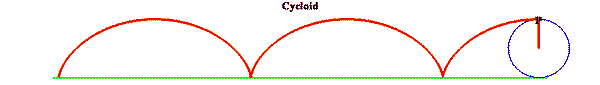
|
Trochoid
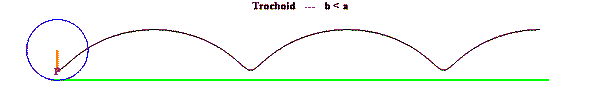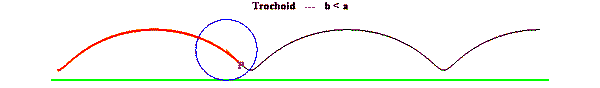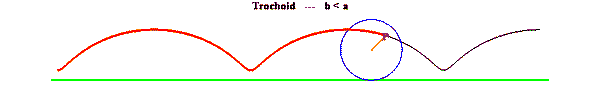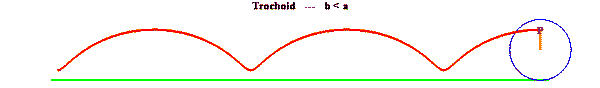


|
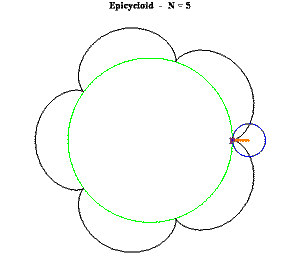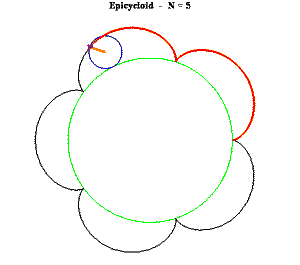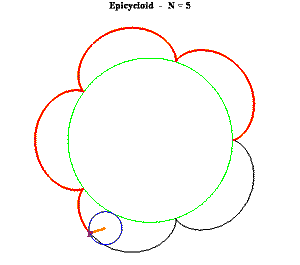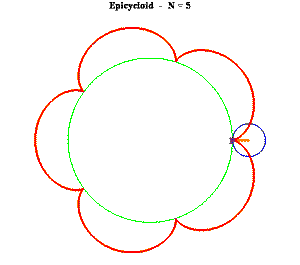
Epicycloid
"Epi" implies the trace of a point on a circle rolling outside another circle . . .
. .
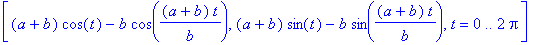 x x
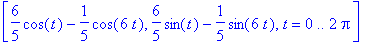
|
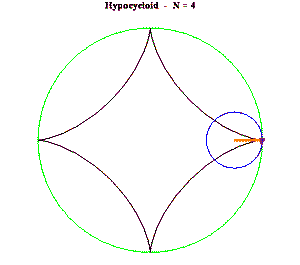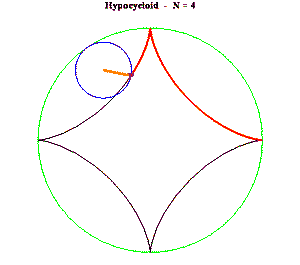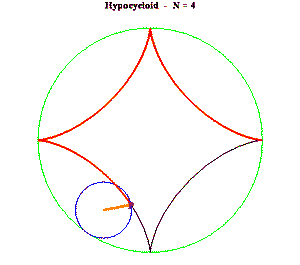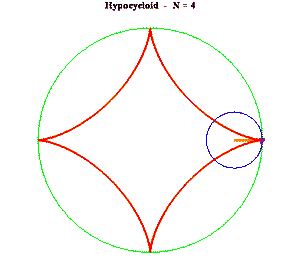
Hypocycloid
. . . .while "Hypo" implies the trace of a
point on a circle rolling inside another
circle . . . .

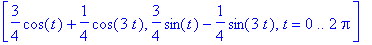
|
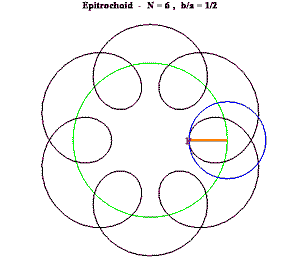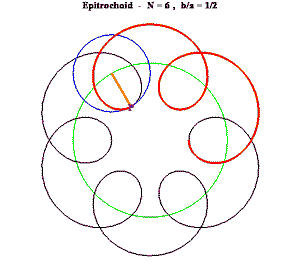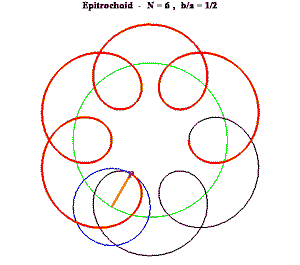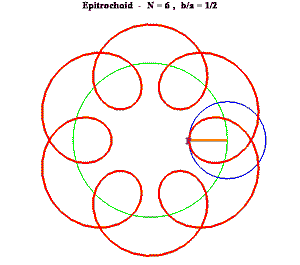
Epitrochoid
.................... and "tro" implies looping rather
than a cusp.
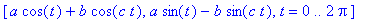

|
Hypotrochoid

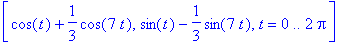



|
Dr. Chabot's Maple Work Sheets can be altered to graph
any curve in the cycloid family on any domain. However, you must
own a copy of the Maple software.
|
Arguably, the Cycloid Family of
curves features the most distinguished group of investigators in
mathematics.
Galileo and Father Mersenne are credited with being the first to name
and discuss its special properties (1599). They were
followed
by Torricelli, Fermat, Descartes, Roberval, Wren, Huygens, Desargues,
Johann Bernoulli, Leibniz, Newton, Jakob Bernoulli, L'Hôpital and
others.
This is probably too brief a list.
One might assert that a
fascination with the motion of the cycloidal curves led a century of
civilization's greatest mathematicians into modern mathematics.
Certainly, the
birth of the calculus, especially the calculus of variations,
flourished
among these remarkable men who were determined to understand its many
special
qualities.
Because of the frequency of
disputes among mathematicians in the 17th century, the cycloid became
known as
the "Helen of Geometers."
The name is appropriately based on Greek
mythology.
Helen was the most beautiful woman in the world. The Trojan war
that followed her capture was one of the fiercest conflicts in ancient
times.
|
| Shikin, Eugene V., Handbook and Atlas of
Curves, CRC Press, 1995.
Yates, R. C., Curves and their Properties,
NCTM, 1952. Also in A Handbook on Curves and their Properties,
various publishers including the NCTM.
Weisstein, Eric. W., CRC Concise
Encyclopedia of MATHEMATICS, Chapman & Hall/ CRC, 2nd ed., 2003.
|
For Mathematica® code that will create many of
these graphs:
Gray, A., MODERN
DIFFERENTIAL GEOMETRY of Curves and Surfaces with
Mathematica®, 2nd. ed., CRC Press, 1998.
< http://mathworld.wolfram.com/Cycloid.html >
|
|