Curve Bank Home

and for arc length . . . .
. 
 

There are several methods for drawing a lemniscate.  The easiest is illustrated above.  Draw a circle and then extend a diameter to become a secant.  The center of the lemniscate  O  will be  times the radius of the circle.  Through  O  draw several segments cutting the circle.  The pattern of the lemniscate emerges in the first quadrant.
 
 

For the more mechanically minded, we suggest using the method described in E. H. Lockwoods' book. Try building a lemniscate "machine."

Solution

This problem is found in many calculus texts.
 
 

The mathematical lineage in Basel is amazing.  James taught his brother John.  John taught L'Hôpital the famous rule, but John's best known student was another native of Basel, Leonhard Euler.   Daniel Bernoulli would earn 10 prestigious awards from the French Académie Royale des Sciences, a record only matched by Euler.      His cousin, Nicholas Bernoulli, was the first to pose the famous St.Petersburg paradox.  The chair of mathematics at the University was held by a Bernoulli for over a hundred years (1687 - 1790).

Today, the Basel phone book lists many Bernoullis and family members are still on the faculty of the University.

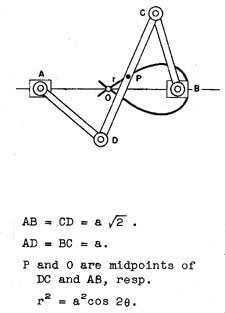
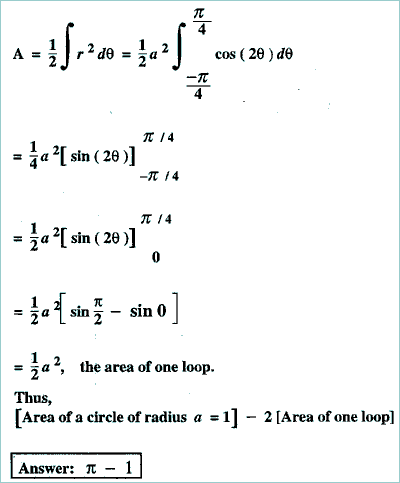
In 1694 James Bernoulli (left) published a curve in Acta Eruditorum that he described as being  "shaped like a figure 8, or a knot, or bow of a ribbon."   Following the protocol of his day, he gave this curve the Latin name of lemniscus, which translates as a pendant ribbon to be fastened to a victor's garland.  He was unaware that his curve was a special case of the Ovals of Cassini. 

His investigations on the length of the arc laid the foundation for later work on elliptic functions.  But his most important contributions were in probability.  We still use the terms "Bernoulli trials" and "Bernoulli numbers" as first suggested in his great classic ArsConjectandi. For example, he included the Bernoulli number of the sum of the 10^th powers of the first 1,000 integers to be

He wrote that he calculated this in "half of a quarter of an hour."

James joined with his younger brother, John (right), in recognizing the importance of Leibniz's highly abbreviated analysis of infinitesimals.  In the late 1600s this trio produced almost all of what we now call elementary calculus as well as the beginnings of ordinary differential equations.  John contributed the name "integral calculus."

John's name is also associated with two other famous curves, the brachystochrome and the catenary.  Unfortunately, he is also remembered for an arrogant personality and the harsh treatment of his son, Daniel.  Daniel won an important prize given by the French Academy, a prize his father thought he should have received.

A fourth Bernoulli, Nicholas, was the first to state the St. Petersburg paradox.  He was also a nephew of James.  Today we experiment with this paradox as Buffon's Needle, using computer and graphing calculator programs.

The Bernoulli family of distinguished mathematicians and scientists is virtually synonymous with the city of Basel in Switzerland.      Though at times dysfunctional in centuries past, the family has remained a significant contributor to the life of the University.  The Bernoullis are our most important mathematical dynasty. 

Formulas and more calculations:
http://mathworld.wolfram.com/Lemniscate.html

Applet:
http://iaks-www.ira.uka.de/home/egner/linkages/lemnis.html
 

Gray, A.,  MODERN DIFFERENTIAL GEOMETRYof Curves and Surfaces with MATHEMATICA®, 2nd. ed., CRC Press, 1998.

Lockwood, E. H.,  A Book of CURVES, Cambridge at the University Press, 1961, pp. 111-117.

Yates, R. C.,  Curves and their Properties, NCTM, 1952, pp. 98 - 99.  Also in A Handbook on Curves and their Properties, various publishers including the NCTM.

Weeks, C.,  Daniel Bernoulli Exhibition, University of Basel, May,2000.  The British Society for the History of Mathematics, Newsletter 42, Winter 2000, pp. 6-8.