| Back
to . .
.
 Curve
Bank Home Curve
Bank Home
 Curve
Index Curve
Index
Deposit #98
Robert G. Chester
Intellectual
Property Associates
Tumwater, WA
N914TJ@comcast.net
____________
Good
visual imagination of motion in more than one plane may be
enhanced by first thinking about the following:
Equatorial orbit . . .

combined with a polar orbit:

Follow the red point/circle in both animations,
The movies have been updated from the .mov format to the .mp4 format.
|
|
|
|
|

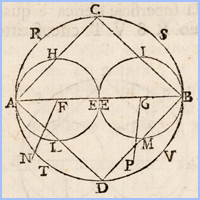
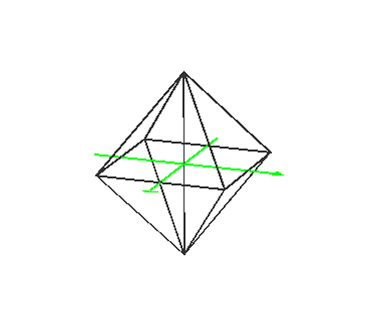
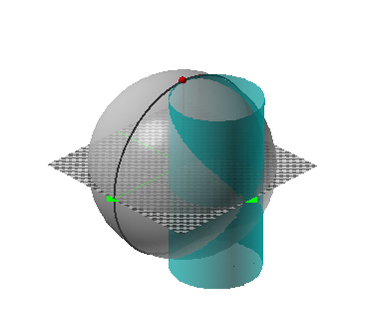
Quasi-Spherical Orbits and Surfaces
"Life of a Point under Dual
Rotations"
Did you know . . .
A Quasi-Spherical Orbit results from the simultaneous rotation of a point
about two or more axes with a common center. Initially, an
individual might use a
mental model of the dual motions of our Earth. Visualize the spin
the Earth on a daily basis of 24 hours while simultaneously moving
about the sun in a 365 day cycle. Though easy to understand, this
mental model is not completely accurate. While the Earth rotates
simultaneously on at least two axes, the axes of the rotations do not
intersect; there is no common center of rotation. A "QSO"
must have a common center of mass for all rotations. Moreover,
there is nothing that says the rotation has to be circular, or even on
a unit sphere. A heliocentric model is merely a simplication to
help the viewer's
initial imagination.
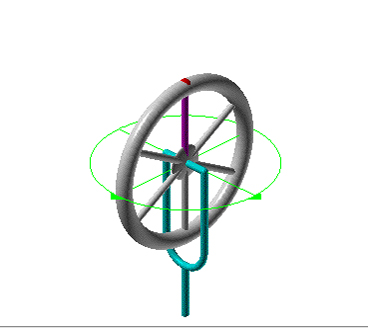
Chester uses a unicycle to model
rotation around the fork of two planes while the wheel itself is also
in motion on the exterior about the axis. He has produced an exhaustive study of
the "life of a point" in both planar and space curves. Obviously, the mathematics of relative
speed and position is often quite complex. View the attached "pdf" file for the
unicycle as a sampler.
References
|
Chester, Robert G, QSO - the Mathematics and
Physics of Quasi-Spherical Orbits, Intellectual Property
Associates, 2009.
ISBN 978-0-9840727-0-5
See Robert Chester's YouTube streaming
video.
|
Fuller, R.
Buckminster,
http://www.bfi.org/our_programs/who_is_buckminster_fuller/design_science/dymaxion_map/the_fuller_projection_map
|
Gray, Alfred, Modern Differential Geometry of Curves
and Surfaces with MATHEMATICA®, 2nd ed., CRC Press, 1998, pp. 927,
933.
|
Simpson, Andrew, Pursuit Curves for the Man in the
Moone, The College Mathematics
Journal, vol. 38, no. 5
(2007), pp. 330-338.
|
| Pae, Peter, Aviation: Taking a ride on a
'perfect flight,' Los Angeles
Times: Business,
September 13, 2007, p. C1. |
|
|





 2009
2009