Tautochrone

Back to . . .

The Tautochrone

The classic simple plane curve first investigated by Christian Huygens (1629 - 1695)

Click on the above play button (right arrow) to replay the animation.

This section: MATHEMATICA® . . .

Def: The solution of the Tautochrone is the determination of the type of curve along which particles move, subject

to a specified force,to arrive at a given point in the same time interval no matter from what initial point it starts.

Def: The cycloid is the locus of a point on the circumference of a circle
where a circle of radius a rolls along a fixed straight line.

The essential cycloid equations:

Many famous mathematicians
have investigated the cycloid and its tautochrone.

Finding the tautochrone was related to the cycloid, Huygens challenged others to investigate its properties.

It is fun to guess how Huygens, Newton, Jean Bernoulli, Euler, Lagrange and even Herman Melville (1851) would have reacted if they had been able to see how easily contemporary students can display and animate "their" tautochrone.

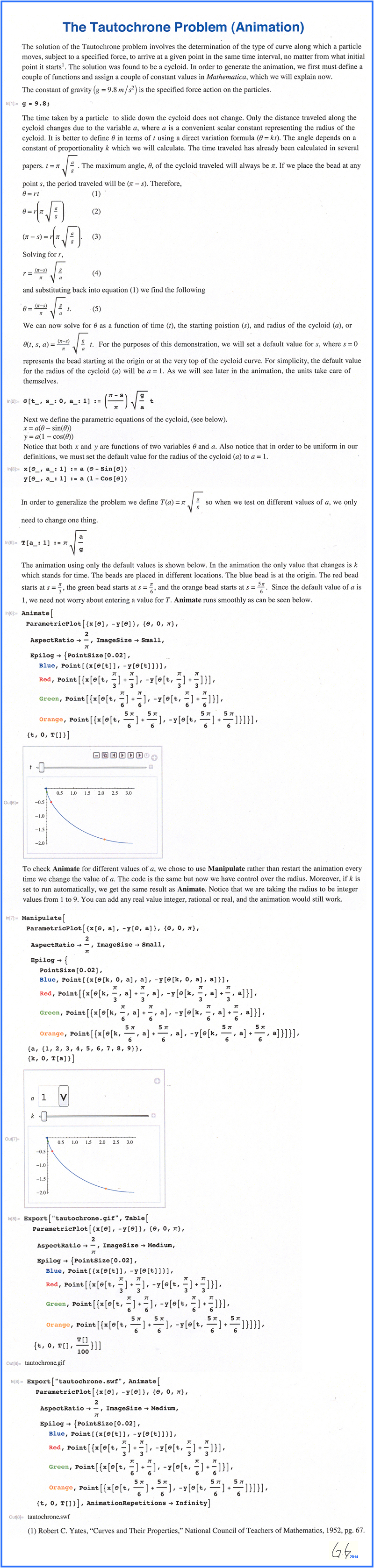
The Figures on the left are from Huygens' Horologium oscillatorium (1673).

Notice FIG. II would result in a
clock pendulum swing of the cycloid.

Physicists and mathematicians applaud Huygens' work on the pendulum. He recognized its path to be that of the much studied cycloid. For this reason, the cycloid is sometimes called the tautochrone.

Huygens also investigated refraction of light in crystals. This was to become an enormous field in chemistry.

Though brief, his De Ratiociniis in Aleae Ludo or On the Calculations in Games of Chance (1657) is considered the first text written on probability.

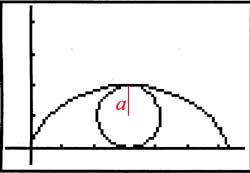Cycloid as an inverted "try-pot" from Moby Dick.

The tautochrone and Herman Melville

Click on the image
for a larger view.

Melville wrote that his time at sea had served as his "Harvard" and his "Yale." He seemed anxious to prove that he had learned mathematics to the extent that he was familiar with the properties of the cycloid. In particular, the three fiery "try-pots" on the deck of the Pequoc, for rendering oil from the blubber of a whale, would illustrate the geometrical properties of a tautochrone when the soapstones used for cleaning rolled from side to side. Moreover, he noted the try-pods were always kept clean and polished when not in use.

Reproduced from the first edition.

The NCB thanks the Huntington Library, San Marino, California, for permission to reproduce this text as it appears in the first edition of Herman Melville's American classic, Moby Dick (1851).

We also gratefully thank the Huntington for permission to reproduce Hugens' illustrations from the Horologium oscillatorium (1673).