Volume of a Box and Exponential Regression

Back to . . .

Deposit #120

James Metz
Kapi'olani Community College
Honolulu, HI

metz@hawaii.edu

The Classic Volume of a Box Problem
. . . extended to

Exponential Regression

Note: On the left the boxes are arranged by the cube
of the even integers from 8 to 24.

On the right is the cost of each box from $2.50 to $7.85.

This same set of data may be used as an Excel exercise.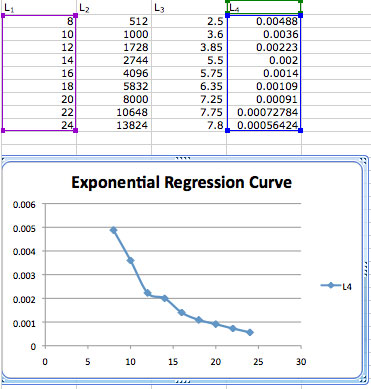

A Comparison of the two graphs invites closer inspection of the data. Note the increment between $3.60 to $3.85 and $5.50 to $5.75 is the same, but also the smallest of the entire data set. This produces a "kink" in the curve.

The graphing calculator was programmed to produce a smooth curve; whereas "Excel" simply connects the points. Interesting!

For more ideas and activites see the following:
Coll, Davis, Hall, Magnant, Stankewicz and Wang, "Integer Solutions to Box Optimization Problems," The College Mathematics Journal, MAA, Vol. 45, No.3, May, 2014, 180-190.

A quick and interesting graphing calculator
application of exponential regression.

Virturally all students in advanced algebra and calculus have solved a "volume of the box" problem. This activity uses boxes and a graphing calculator to illustrate exponential regression.

     L1 is the list of one dimension of each box.
     L2 is the cube of L1, or the volume of each box.
     L3 is the cost of each box.

L4 is the average cost per cubic inch or L2/L3.

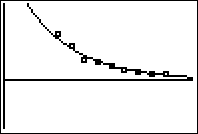
After plotting the points ( L1, L4 ) . . . , and noting the curve,

we determine the exponential regression equation.

2012.