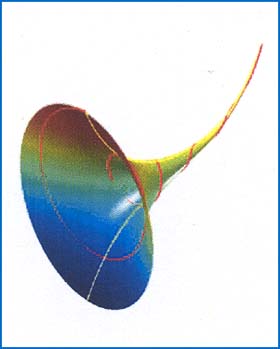
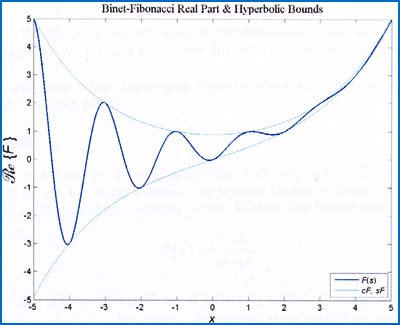
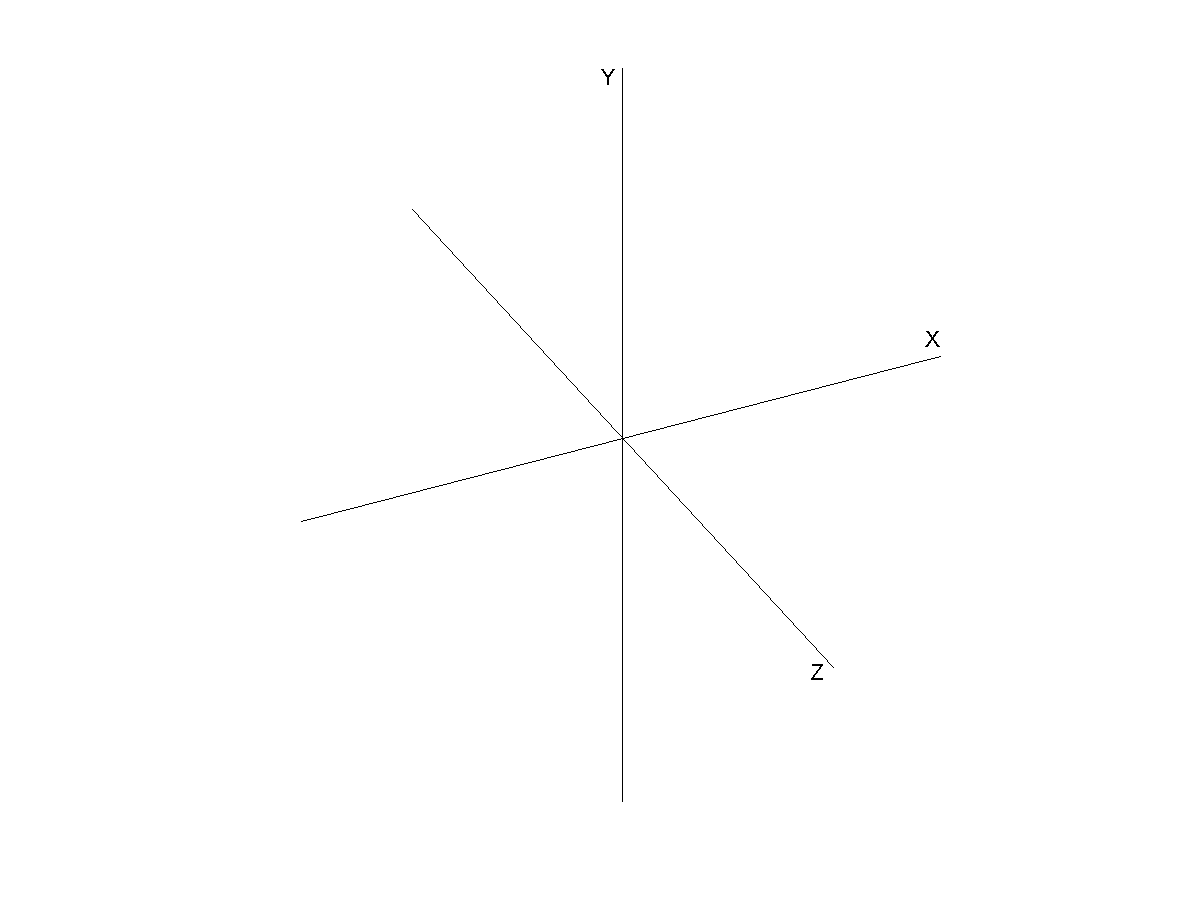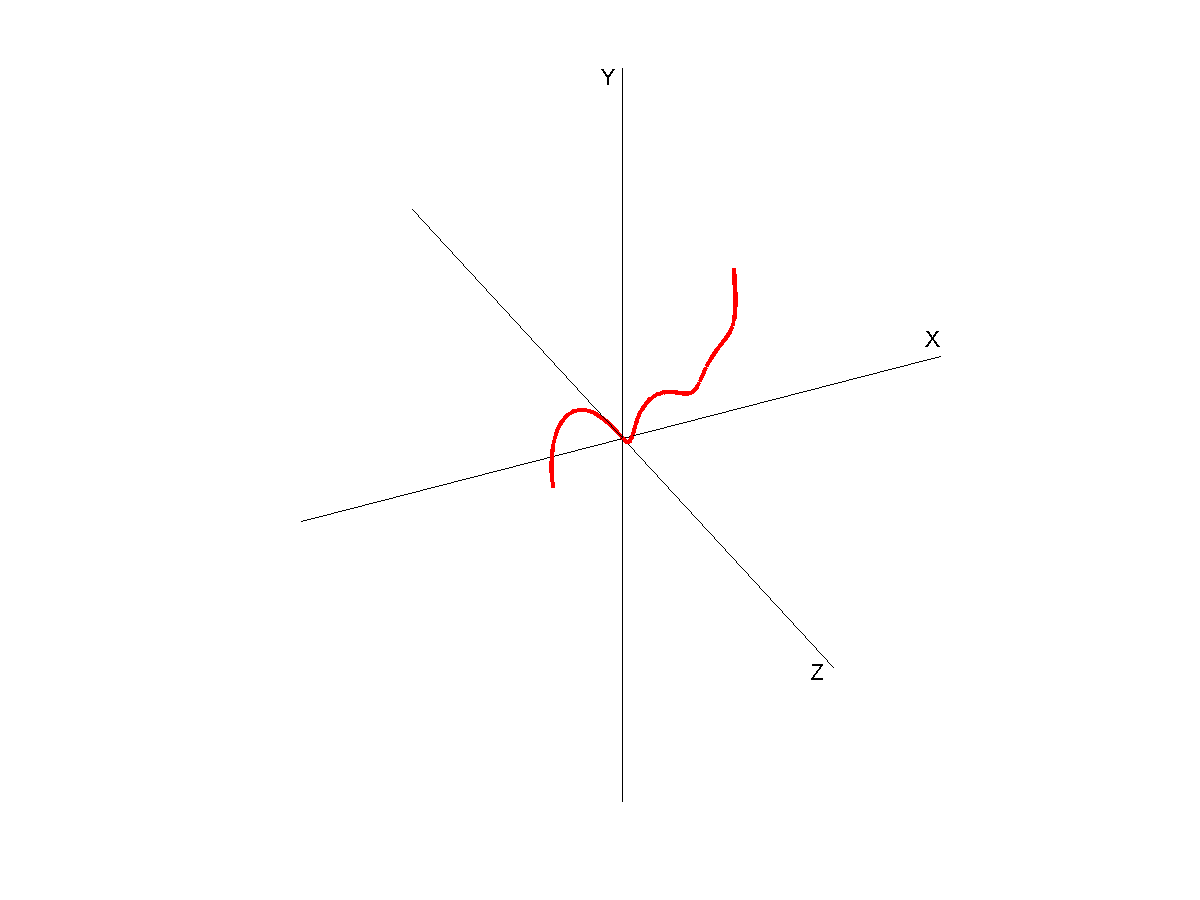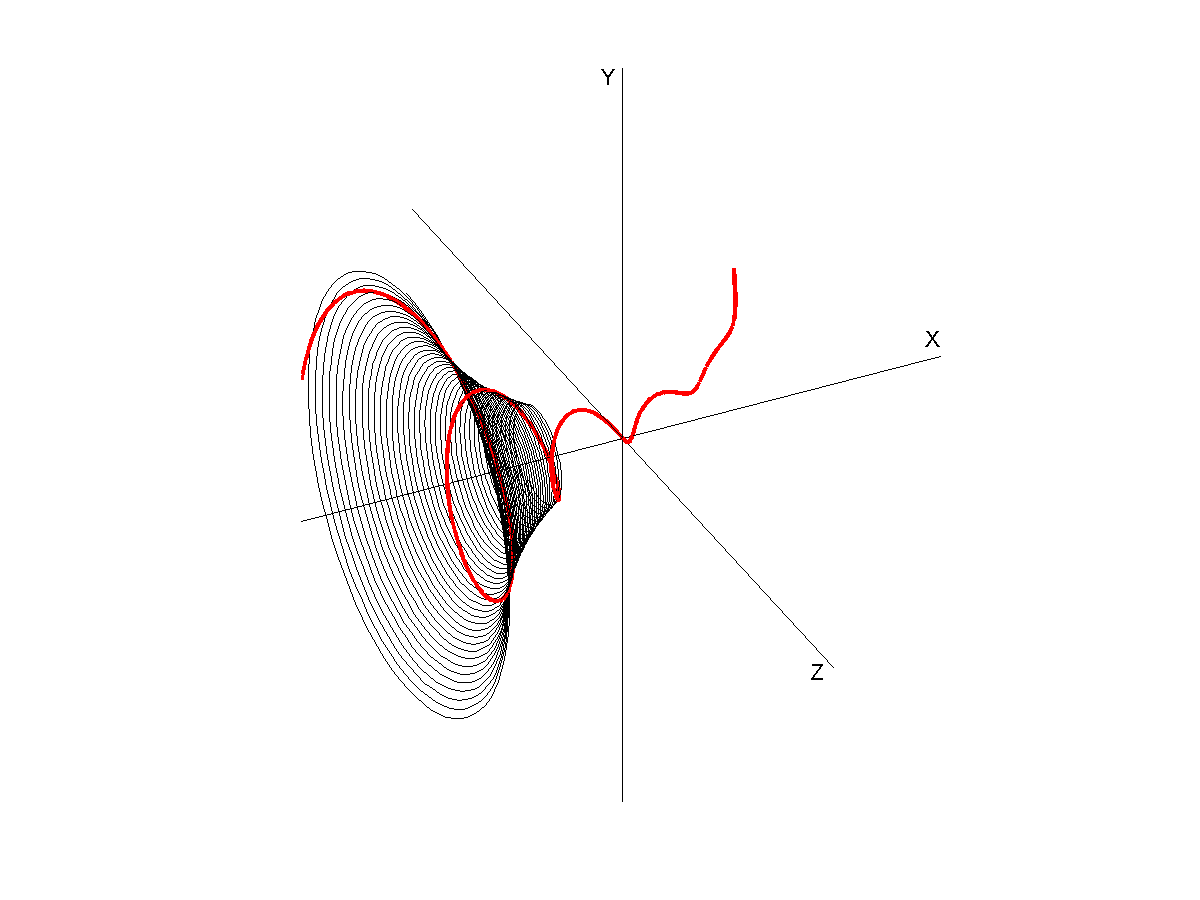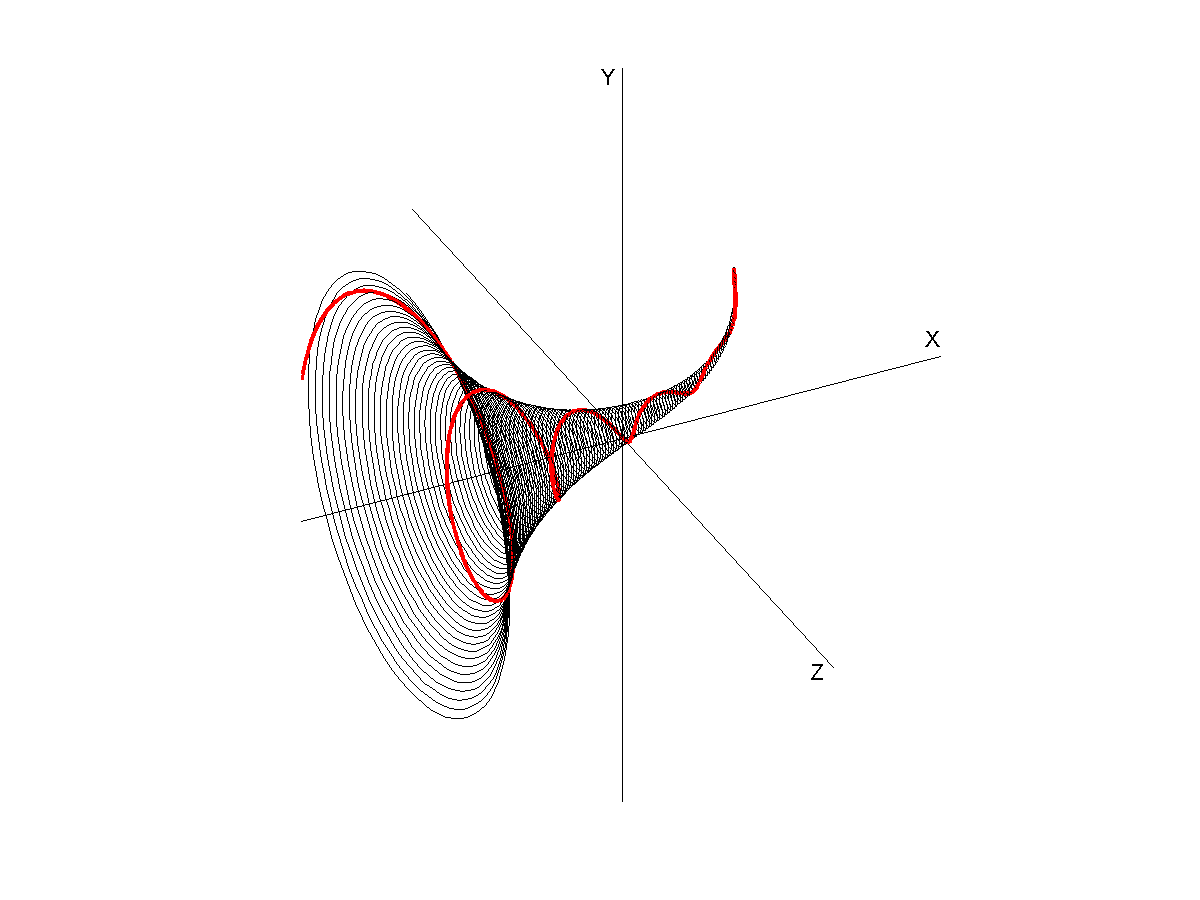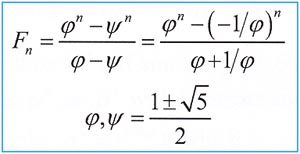The Fibonacci Spiral and Pseudospirals

Back to . . . .

Curve Bank Home
Curve Bank Index

NCB Deposit # 129

Dr. Cye Waldman
cye@att.net

The "Other" Fibonacci Spiral and Binet Spirals

The Golden Shofar or Horn of Plenty

The "Other" Fibonacci Spiral, Binet Spirals and Golden Shofar Horn of Plenty.
Click here for the full article including equations and Matlab code.

The classic Fibonacci spiral is composed of quarter-circular arcs that grow as the Fibonacci sequence. However, this is a pseudospiral in the sense that the curvature does not grow monotonically. The spiral is based solely on the Fibonacci sequence and no other mathematical functions are involved. The defining quality of the "Other" spirals is that they occur for negative values of the argument with the x-axis crossings being equal to the (negative) sequence.

Waldman explores the spiral in the entire complex plane and then moves to a three-dimensional curve in space by graphing the imaginary component of the function on a perpendicular plane. He thus represents the "Other" Fibonacci planar spiral as a three-dimensional curve in space.

"It would be surprising if this had never been done before, and indeed it has. We were seeking a new class of spirals from the Binet formula, but alas, the best laid schemes . . ."

Dr. Cye Waldman

Appropriately for the Fall season, the three-dimensional spiral symbolically represents a Horn of Plenty or a Golden Shofar (Stakhov and Rozin). Translating from the Hebrew language, "Shofar" means horn, traditionally a ram or antelope horn; it is used ceremoniously on the Jewish New Year and Day of Atonement (Yom Kippur).

The "pdf" file provides extensive Matlab code for your browsing pleasure.
A sampler of the Shofar-Horn of Plenty code is as follows:

References

A large number of useful references and links are listed at the close of the "pdf" file.

Other Waldman contributions to the NCB:
Sinusoidal Spirals: < ..//waldman/waldman.htm >
Bessel Functions    < ..//waldman2/waldman2.htm >
Gamma Funcions   < ..//waldman3/waldman3.htm >
Polynomial Spirals and Beyond   < ..//waldman4/waldman4.htm >
Fibonacci and Binet Spirals with a touch of Mondrian < ..//waldman6/waldman6.htm >

The NCB thanks Dr. Waldman for his strong contibutions.

Other spiral Deposits in the NCB:
< ..//spiral/spiral.htm >
< ..//log/log.htm >

Other Fibonacci, Liber Abaci, and Pisa Deposits in the NCB:
< ..//fibonacci/fibonacci.htm >

b. Feb. 2, 1786
Renne

d. May 12, 1895
Paris

Jacques Philippe Marie Binet is linked to Fibonacci and the golden ratio by the following Binet function:

Moreover, others go so far as to suggest Binet might have been the first to have formulated matrix multiplication. Traditionally, this operation is credited to Cayley (1821-1895) who was far younger. In addition Binet overlapped in time and place with Cauchy (1789-1857) and shares credit with Cauchy for the Cauchy-Binet formula.

2013