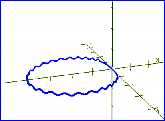
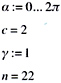The shell as a
curve and a surface.
. . . and to plot,
set the parameters as . . .
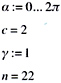
___________________
All animations were created using
the Grapher program in MacOS
X.
Mac OSX viewers can open
Zettler's shell animation in a freeware program. The
surface can be rotated using the Grapher tool. Simply
click on the graph and move the mouse. (In the Applications folder on the hard
drive, find the Utilities
folder. Download the free
Grapher to
the
desktop.) Download the
attached file to your desktop, unzip the file and then open in Grapher.
Related Curves
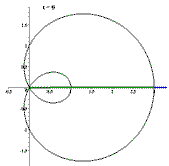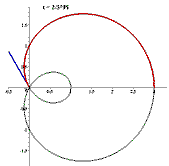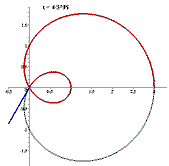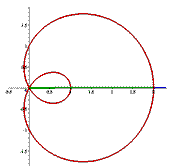
Limaçon
|
|
|
 Deposit # 83
Deposit # 83
| Back to . . .
 Curve
Bank Home Curve
Bank Home
 Curve
Index Curve
Index
Sea Shells
as
Mathematics
"Recently I studied a shell
collection. The beautiful shapes fascinated me and made me search
for a mathematical model. I found a rich and time-honored
history."
Dr. Thomas Zettler
Munich, Germany
|
This section . . . .
Larger
Animation
|
The shell surface is
given by
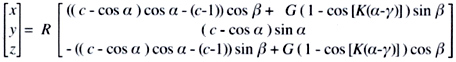
where 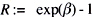 is the
radius growth function. is the
radius growth function.
|
For
plotting the shell surface . . .

|
The second term (G) forms the
grooves of the shell.
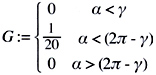
while gamma ( γ ) separates two
angular
segments, one with and the other without
grooves.
In the K
portion of the equation, the number of grooves is defined by n:
|
Shells
have a venerable history. Pappus ascribes their
"invention" to Nicomedes (ca. 240 BC). Later in the seventeenth
century, the conchoid was a favorite specimen for the new
methods of analytical geometry and calculus. Today, we find
shells, conchoids and limaçons are popular for those
experimenting with computer graphing software and graphing calculators.
Note the first term in the
first line of the initial equation is  .
This is a
special case of Pascal's conchoid, better know as the limaçon
(from the Latin word for "snail," limax,
and was discovered by Etienne Pascal (1588-1640), father of the famous
Blaise Pascal. .
This is a
special case of Pascal's conchoid, better know as the limaçon
(from the Latin word for "snail," limax,
and was discovered by Etienne Pascal (1588-1640), father of the famous
Blaise Pascal.
|
The limaçon was in fact
constructed before Pascal by the famous artist Albrecht
Dürer. Due to the appearance of lines he used in the
construction, Dürer called the image a "spider curve."
Albrect Dürer,
Unterweysung
der Messung mit dem Zirkel und Richtscheyt, 1525
(The
Painters' Manual, Abaris Books, NY, 1977)
A brief list of printed
sources
that should
be in most university libraries.
|
Gray,
Alfred, Modern Differential Geometry of
Curves and Surfaces with MATHEMATICA®, CRC Press,
1998, p. 72.
|
| Lockwood,
E. H., A Book of CURVES,
Cambridge Unniversity Press, 1961, pp. 127-129. |
Weisstein,
E. W., CRC Concise Encyclopedia of Mathematics, CRC Press,
1999, pp. 499-500. See Dürer's Conchoid etc.
|
| Yates,
R. C., Curves and Their Properties. NCTM, 1952,
pp. 31-33, etc. |
|
The
National Curve Bank
thanks Dr. Thomas Zettler of Munich, Germany for Deposit # 83.
Dr. Zettler created these
animations using GRAPHER running on Macintosh MAC-OS X.
thomas.zettler@arcor.de
|
|
|


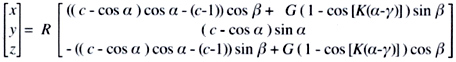
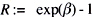 is the
radius growth function.
is the
radius growth function.
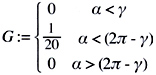
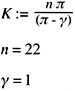
 .
This is a
special case of Pascal's conchoid, better know as the limaçon
(from the Latin word for "snail," limax,
and was discovered by Etienne Pascal (1588-1640), father of the famous
Blaise Pascal.
.
This is a
special case of Pascal's conchoid, better know as the limaçon
(from the Latin word for "snail," limax,
and was discovered by Etienne Pascal (1588-1640), father of the famous
Blaise Pascal.