Man in the Moon Pursuit Curve

Back to . . .

Curve Bank Home

Classics Index Page

Deposit #102

Andrew Simoson
King College, Tennessee

Gary Brookfield
CSULA

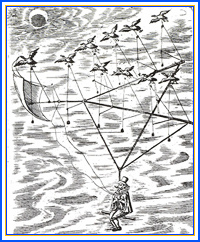
The Family of Pursuit Curves

"The Man in the Moone or A Discourse on a Voyage Thither"
Francis Godwin - 1638

frontissmall

Swan's Speed < Moon's Speed	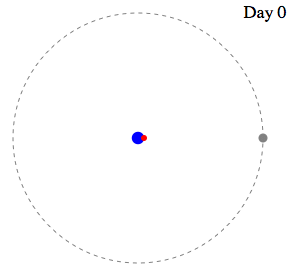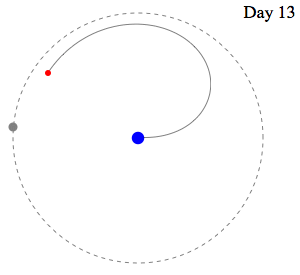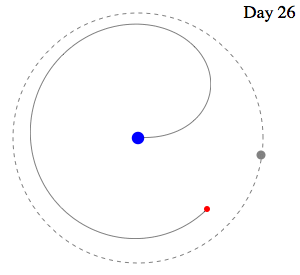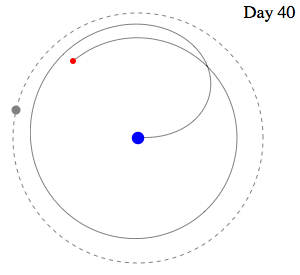Swan's Speed = Moon's Speed	*What is a "Pursuit Curve?"* One particle travels along a specified curve, while a second pursues it, with a motion always directed toward the first. The velocities of the two particles are always in the same ratio. Thus, the two beads move with related velocities. When the ratio k of the two velocities is greater than one ( k > 1 ), the pursuer travels faster than the pursued. The question then becomes, "At what point do the two meet?" What is the "capture" point?
Swan's Speed slightly greater than Moon's Speed leads to capture. . .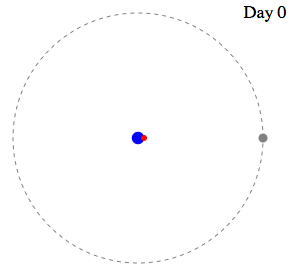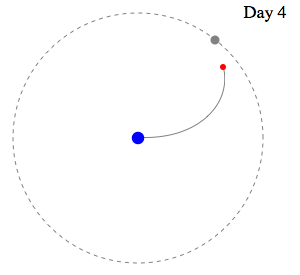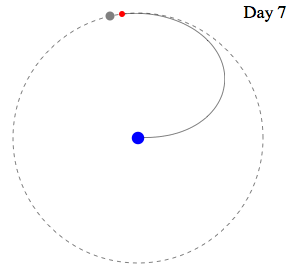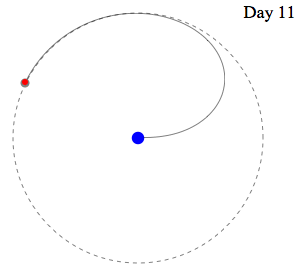	And an even faster capture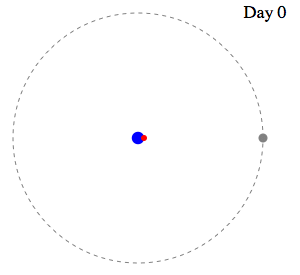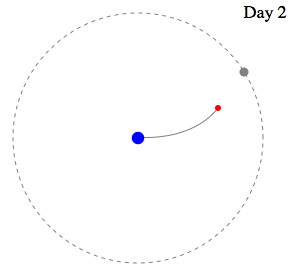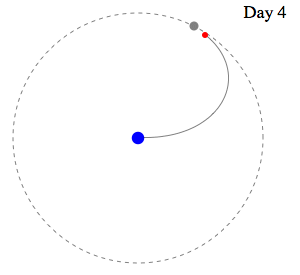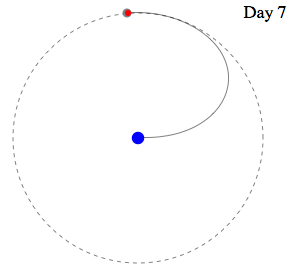

Historical Sketch:

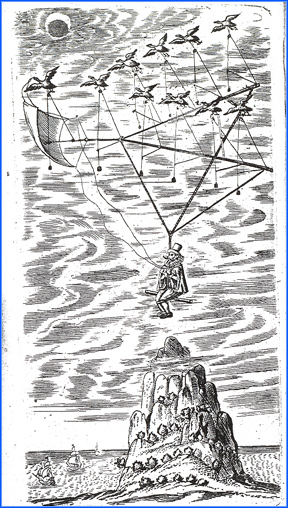frontispiece

The First Story in English Literature of Space Travel

Over 300 years before Neil Armstrong stepped on the moon, a bishop of the Anglican church wrote the first English language science fiction tale of the voyage. The story involved a flight to the moon by an astronaut named Domingo Gonsales, the "speedy messenger," in a space "Engine" powered by "wild swan" having "one foote with Clawes, talons and pounces, like an eagle", and the other being "on the whole like a swan or water fowle." He affectionally named his fictional birds "Gansas" with "gansa" being the German name for "goose." His Gansas migrated in much the same pattern as geese.

"Not many hours after the departure . . ., my Gansas began to bestir themselves, still directing their course toward the globe or body of the Mooon, and they made their way with that incredible swiftness, as I think they gained not so little as fifty Leagues in every hour.

In The Man in the Moone Godwin clearly foreshadows the great scientific discoveries of the 17th century, including the rotation of the earth in its orbit about the sun and the law of gravity.

"Whereby it appeareth, not only that my Gansa's took none other way than directly toward the Moon, but also, that when we rested (as at first we did for many hours), either we were insensibly carried, (for I perceived no such motion) round about the Globe of the Earth, or else that (according to the late opinion of Copernicus,) the Earth is carried about, and turneth round perpetually, from West to East. . . ."

(On looking back toward Earth)

". . .the farther we went, the less of the globe of the Earth appeared to us, whereas, . . . the Moon showed herself more and more monstrously huge. . . .On the Moon we discerned certain spots or clouds, as it were, so did I then in the earth. But where as the form of those spots in the Moon continue constantly one and the same, these (spots) little & little did change every hour. The reason thereof I conceive to be this. That whereas the Earth, according to her natural motion, ( for that such a motion he hath, I am now constrained to join in the opinion with Copernicus), turneth round upon her own axis every 24 hours from the West to the East. . . ."

The Earth was . . . "no other than a huge Mathematical Globe, leisurely turned before me, wherein successively, all the countries of our earthly world within the compass of 24 hours. Philosophers and Mathematicians, I . . . now confess the wilfulness of their own blindness. They have made the world believe hitherto, that the Earth hath no motion."sage in this volent flight, I perceived that we began to approach near unto another Earth, if I amy so call it, being the globe or very bopais volent flight, I perceived that we began to approach near unto another Earth, if I amy so call it, being the globe or very body of that star we call the Moon."

Godwin's mathematics included having the birds fly at a constant rate on a nonlinear outward trajectory of more than 11 days. The return to Earth took 8 days but followed a straight line. Modern history of mathematics students will marvel at the accuracy of Godwin's speculative knowledge of science in 1638. His story published after Columbus and Kepler, but before much of Galileo's publicity, includes specific references to Copernicus and no mention of Newton who was yet unborn. Godwin wrote he lived in the "Age of Discovery."

The landing . . .

Moonlanding text

"After eleven days passage in this violent flight, I perveived that we began to approach near unto another Earth, if I may so call it, being the Globe or very body of that star which we call the Moon. I perceived also that it was covered for the most part with a huge mighty sea . . . ."

"The first difference that I found between it and our earth, was, that it showed itself in its natural colors. Ever after I was free from the attraction of the Earth, whereas with us, a thing removed from our eye but a league or two, begins to put on that lurid and deadly color of blue."

"How often did I wish myself . . .that freely I might fill the world with the fame of my glory and renown."

Bishop Godwin

Francis Godwin (1562-1633)
Bishop of Llandaff and Hereford

Andrew Simoson of King College in Tennesse recognized this story provided a great opportunity to introduce pursuit curves and MATHEMATICA® animations to entice modern students to think about space travel. In other passages Godwin's mathematics included having the geese fly at a constant rate on an outward trajectory that was not linear and lasted 12 days.

Important Reference Points for an Historic Overview

c. 45 BC	Zeno	The paradox of Achilles' pursuit of a tortoise.
c. 174 AD	Lucian	The True History A comic satire on travel to the moon by sailing on a waterspout.
c. 1300	Dante	The Divine Comedy Beatrice and Dante fall upwards from a mountaintop on Earth to tour the solar system.
1452-1519	Leonardo da Vinci
1543	Copernicus	De Revolutionibus Orbium Coelestium
c. 1620-30	Johannes Kepler	The Dream or Somnium
c. 1630	Galileo	Calculated a ball would fall from the moon to the Earth in3 hours, 22 minutes, and 4 seconds.
c. 1599 1638	Francis Godwin Bishop and Historian (1562-1633)	The Man in the Moone. Published posthumously by a friend. The first story of space travel in English literature. By 1768 at least 25 editions are know to have existed in a wide variety of languages.
1656	Cyrano de Bergerac	Voyage to the Moon
1749	Pierre Bouguer	Figure de la terre déterminée
1943	Antoine de Saint-Exupéry	The Little Prince
July 20, 1969	Neil Armstrong	"One small step for man, one giant leap for mankind."

While still a student in 1593 Kepler wrote a series of speculations on the geography of the moon. Later in 1610, Galileo sent him an early copy of The Starry Messenger. Kepler replied, ". . . for those who will come shortly to attempt this journey (to the moon), let us establish the astronomy; Galileo, you of Jupiter, I of the moon."

The opportunities for animation of pursuit curves are enormous. The NCB invites faculty and students to try their hand at some of these problems as class projects. Then hopefully you will add a "choice" effort to our NCB MATH Archive collection as a sampler of a fun activity from your campus.

Useful Links and Books

Simoson, Andrew J., Pursuit Curves for the Man in the Moone, College Mathematics Journal, 38 (2007) 330-338.

Koestler, Arthur, The Sleepwalkers, Penguin Books, 1959.

Lear, John, Kepler's Dream, U. of California Press, 1965.

< ..//pursuit/pursuit.htm >

< ..//pursuit2/pursuit2.htm >

For more information on pursuit curves: < http://en.wikipedia.org/wiki/Curve_of_pursuit >

For a variety of pursuit problems: < http://mathworld.wolfram.com/topics/ApolloniusPursuitProblem.html >

For the evolute in JAVA: < http://www-history.mcs.st-and.ac.uk/history/Curves/Pursuit.html >

Note: The French scientist Pierre Bouguer attempted to measure the density of the Earth by using a plumb line deflected by the attraction of gravity. He collected data on the top of a Peruvian mountain. While he was more or less unsuccessful, the thought that he would attempt this in South America in 1740 is slightly amazing.

stamp

stamp

Simosonsig

MATHEMATICA^® animations contributed by

Dr. Gary Brookfield

2010.